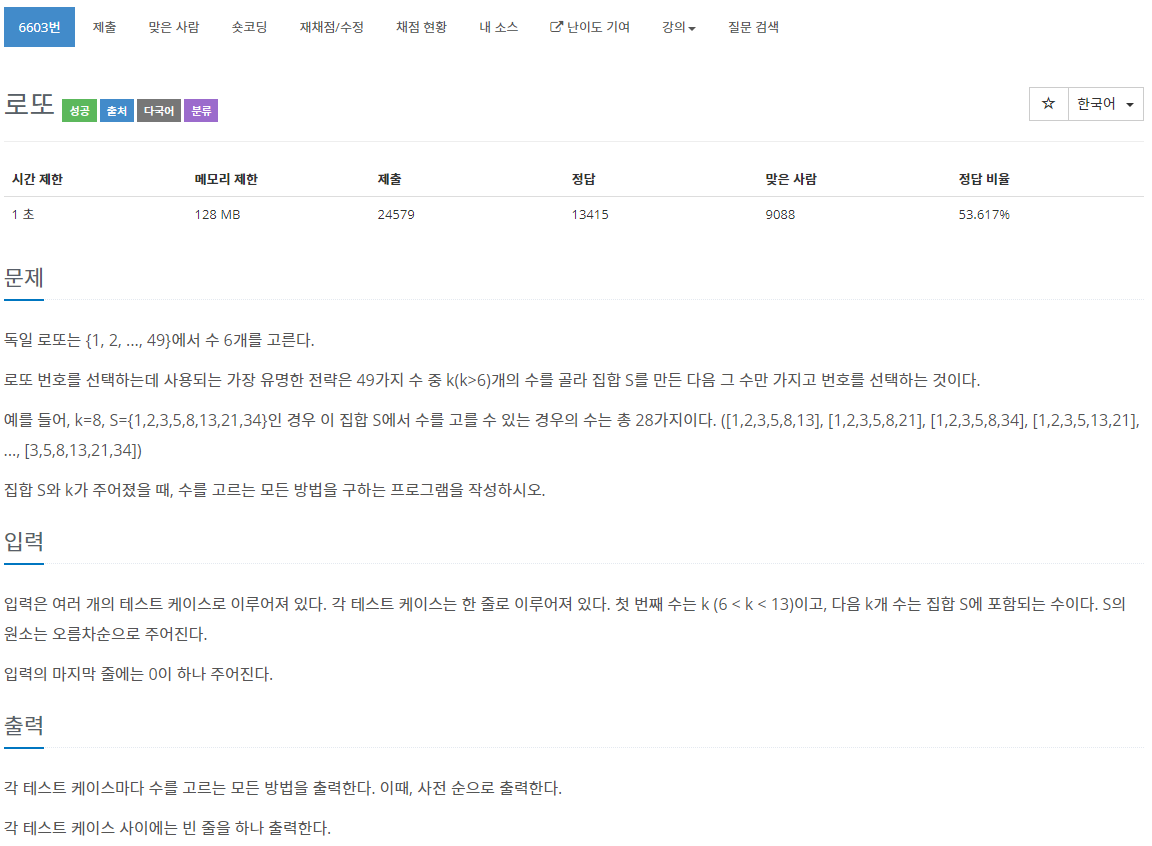
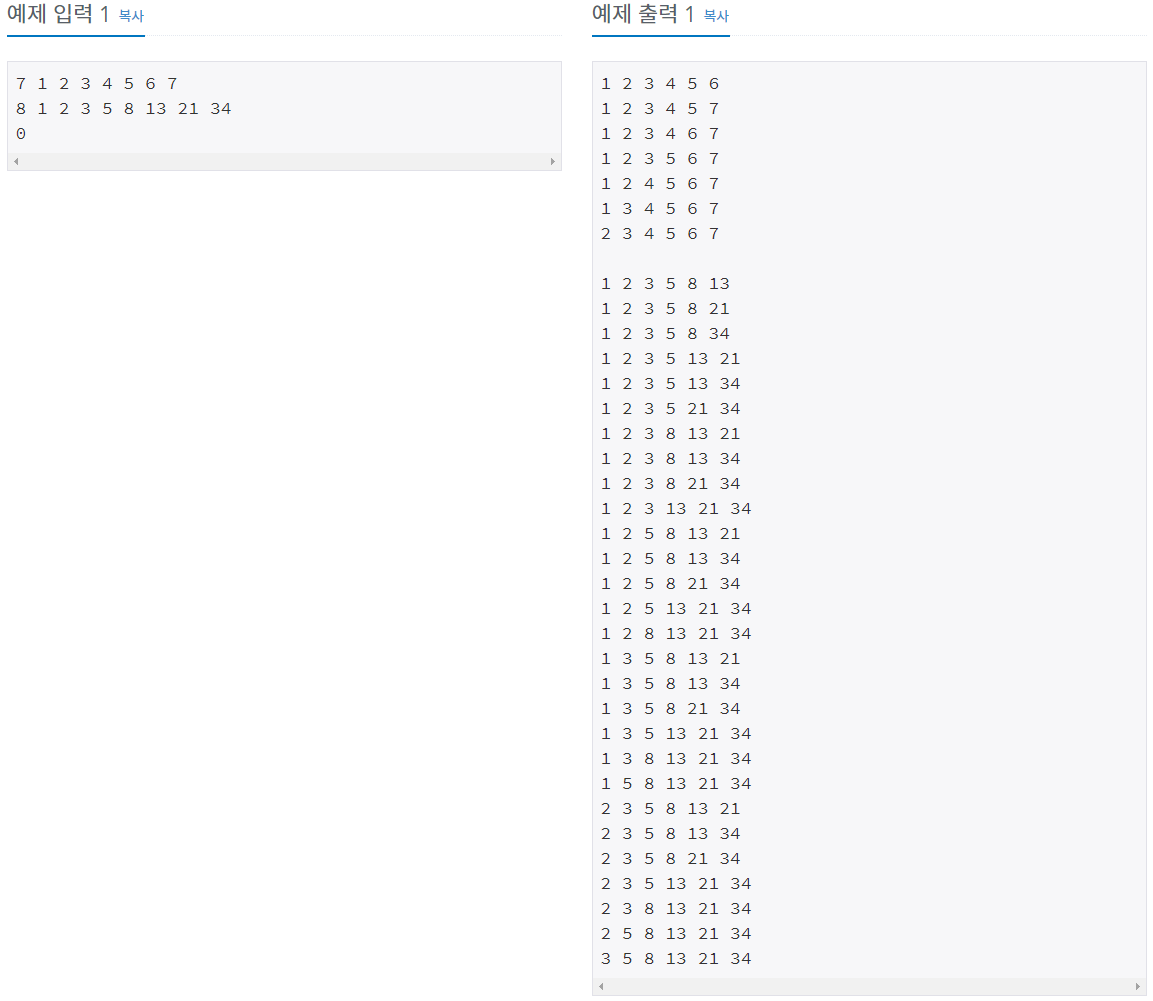
문제
소스코드
|
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
|
#include<iostream>
#include<vector>
constexpr int MAX_SET = 28;
std::vector<std::vector<int>>S;
std::vector<int>V;
int K{};
bool visited[MAX_SET];
void BackTracking(int curr, int index) {
if (curr > 6) {
for (const auto& i : V) {
std::cout << i << " ";
}
std::cout << "\n";
return;
}
for (int i = 0; i < S[index].size(); ++i) {
//방문여부가 false이면 다음값을 체크하자
if (visited[i] == false) {
//넣을려고 할때 앞에 숫자가 더 작으면 패스
if (V.size() != 0) {
if (S[index][i] < V[V.size() - 1])continue;
}
V.emplace_back(S[index][i]);
visited[i] = true;
BackTracking(curr + 1, index);
//BackTracking 이후
V.pop_back();
visited[i] = false;
}
}
}
int main() {
while (true) {
std::cin >> K;
std::vector<int>setLIst;
if (K == 0)break;
for (int i = 0; i < K; ++i) {
int number{};
std::cin >> number;
setLIst.emplace_back(std::move(number));
}
S.emplace_back(setLIst);
}
for (int i = 0; i < S.size(); ++i) {
BackTracking(1, i);
std::cout << "\n";
}
}
|
후기
이 문제를 해결하기 위한 키워드는 백 트래킹이다.
백 트래킹(Back Tracking)이란 깊이 우선 탐색(DFS)의 구조를 기반으로 문제를 푸는 전략으로 탐색을 진행했다가 더 갈 수가 없으면 되돌아가 다른 길을 찾는다고 해서 이름이 붙였으며 주로 재귀 함수로 구현을 한다.
모든 경우의 수를 찾기만 한다면 관련글에 있는 N과 M으로 도 통과할 수 있지만 여기에 추가적으로 오름차순 즉 다음에 나올 수가 앞에 있는 수보다 작으면 안 되기 때문에 25~27번째 줄에 예외를 처리해서 진행을 하였다.
출처 및 레퍼런스
문제 링크: https://www.acmicpc.net/problem/6603
Color Scripter: https://colorscripter.com/
관련 글
[자료구조 알고리즘/탐색 알고리즘(Search)] - 백 트래킹(Backtracking)
[온라인 코딩/탐색(Search)] - [백준] 15649번 N과 M
'온라인 코딩 > 탐색(Search)' 카테고리의 다른 글
| [프로그래머스] 경주로 건설 (0) | 2020.09.26 |
|---|---|
| [백준] 1697번 숨바꼭질 (0) | 2020.09.10 |
| [구름 IDE] 비타알고 시즌2 졸업(★3) (0) | 2020.07.02 |
| [백준] 1620번 나는야 포켓몬 마스터 이다솜 (0) | 2020.06.21 |
| [백준] 2178번 미로 탐색 (0) | 2020.05.22 |